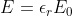What is Dielectric materials?

Dielectric materials are insulating materials that do not conduct electricity. They are used to separate electrical charges and store electrical energy. When an electric field is applied to a dielectric material, it polarizes the material, meaning that its molecules align with the field. This polarization results in an increase in electric capacitance, making dielectric materials useful in capacitors.

Common examples of dielectric materials include ceramics, plastics, and various liquids. The dielectric constant of a material determines how easily it can be polarized and therefore how much it can store electrical energy. Different materials have different dielectric constants, and the choice of dielectric material depends on the desired electrical properties, such as the ability to store energy, the ability to withstand high voltage, and the desired electrical insulation properties.

Gauss’s law in dielectrics

Gauss's law states that the total electric flux through any closed surface is proportional to the charge enclosed within that surface. In a dielectric material, electric flux is proportional to the electric field strength, but the relationship is not linear. The electric field in a dielectric material is reduced by a factor called the dielectric constant. Gauss's law can be written as:

where E is the electric field strength, ρ is the charge density, ε₀ is the vacuum permittivity, and ∇⋅E is the divergence of the electric field. In a dielectric material, the electric field is given by:

where εr is the relative dielectric constant of the material, and E₀ is the electric field in a vacuum.

Dielectric function

The dielectric function is a complex quantity that describes the response of a material to an applied electric field. It is a measure of the ability of a material to store and return electrical energy. The dielectric function is defined as the ratio of the electric displacement field D to the electric field E in a material:

ε(ω) = ε₀ε′(ω) - iε′′(ω) = D/E
where ω is the angular frequency of the applied field, ε₀ is the vacuum permittivity, ε′(ω) is the real part of the dielectric function, and ε′′(ω) is the imaginary part of the dielectric function.

The real part of the dielectric function, ε′(ω), represents the energy stored in the electric field, while the imaginary part, ε′′(ω), represents the energy lost to thermal and other losses. The dielectric function is a complex quantity because it takes into account the frequency dependence of the material's response to an applied electric field.

The dielectric function is important in several fields, including optics, electronics, and electromagnetism. In optics, the dielectric function determines the refractive index of a material and how light propagates through it. In electronics, the dielectric function is used to model the behavior of dielectric materials in capacitors and other electrical components. In electromagnetism, the dielectric function is used to describe the interaction between electromagnetic fields and dielectric materials.